In this article, we will discuss buckling load numerical.
1. Introduction
The maximum limiting load at which the column tends to have lateral displacement / tends to buckle is called Buckling Load. It is also known as Crippling Load.
The buckling takes place about the axis having a minimum radius of gyration or least moment of inertia.
| Pcr = ( π2 EI ) / L2 |
It is Euler’s formula for buckling load.
| End Condition | Effective length | Euler’s Buckling |
| Both ends pinned / hinged | Le = L | Pcr = π2 EI / Le2,Pcr = π2 EI / L2 |
| One end Fixed, other and free | Le = 2 L | Pcr = π2 EI / Le2,Pcr = π2 EI / 4L2 |
| One end fixed, other end hinged / pinned | Le = L / √2 | Pcr = π2 EI / Le2,Pcr = 2π2 EI / L2 |
| Both ends fixed | Le = L / 2 | Pcr = π2 EI /Le2,Pcr = 4π2 EI / L2 |
2. Important Formulas
| 1. Pcr = ( π2 EI ) / Le2 |
| 2. Critical Load = 2 x actual load |
| 3. Moment of Inertia (I) = π ( D4 – d4 ) / 64 |
| 4. Safe Load = Buckling load / Factor of Safety (FOS) |
| 5. E = FL / A Δl |
Δl= Elongated length
| 6. Slenderness ratio (λ) = l / Rmin |
Rmin = Radius of gyration
3. Buckling Load Numerical
1. A solid round bar 60 mm in diameter and 2.5 m long is used as a strut, one end of the strut is fixed while its other end is hinged. Find the safe compressive load for this strut using Euler’s formula. Assume E= 200 GN/m2 and factor of safety 3.
Given,
d = 60 mm = 0.06 m
l = 2.5m
E= 200 GN/m2 = 200 x 109 N/m2
FOS = 3
Le = L / √2 = 2.5 / √2 = 1.77 m
we have,
Buckling Load (Pcr) = π2 EI / Le2
= π2 200 x 109 π ( 0.064) / 64 x 1.772
= 400.8 KN
Safe Compressive load = Buckling load / Factor of safety
= 400.8 / 3
= 133.6 KN
2. A slender pin-connected aluminum column 1.8m length of the circular cross-section is to have an outside diameter of 50 mm. Calculate the necessary internal diameter to prevent failure by buckling if the actual load is applied is 13.6 KN and the critical load applied is twice the actual load. Take E for aluminum as 70 GN/m2.
Given,
L = 1.8 m
Outside diameter (D) = 50 mm
Internal diameter (d) = ?
E = 70 GN/m2
= 70 x 109 N/m2
Actual load applied (P) = 13.6 KN
Critical load = 2 x actual load
= 2 x 13.6
= 27.2 KN
Moment of inertia (I) = π ( D4 – d4 ) / 64
= π ( 0.054 – d4 ) / 64
End condition: both ends pinned
Effective length (Le) = L = 1.8 m
Buckling Load (Pcr) = π2 EI / Le2
27.2 x 103 = π2 70 x 109 x π ( 0.054 – d4 ) / 64 x 1.82
= 564019.2 = 13565246.05 – 2.17 x 1012d4
d4 = 13001226.85/ 2.17 x 1012
d = 0.049m
3. An I section joint 400 mm x 200 mm and 6 m long is used as a strut with both ends fixed. What is Euler’s crippling load for columns? Take Young’s modulus of joist as 200 Gpa.
All dimensions are in mm.
Given,
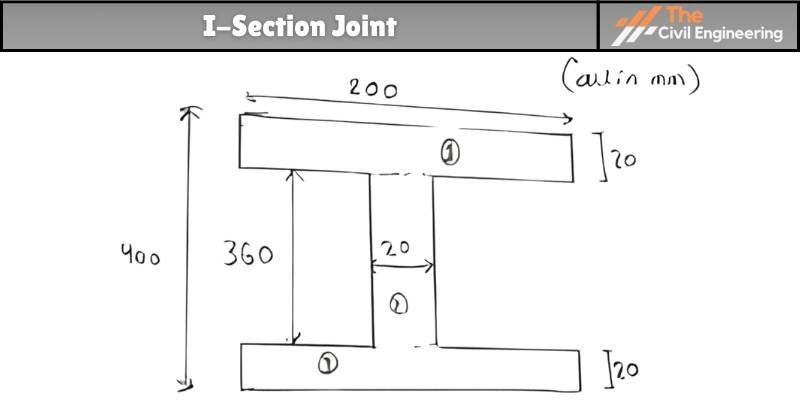
x̄ = 100 mm
ȳ = 200 mm
x₁ = 100 mm x₂ = 100 mm
y₁ = 10 mm y₂ = 200 mm
x₃ = 100 mm y₃ = 390 mm
Buckling Load (Pcr) = π2 EI / Le2
Here, I is Minimum of Iₓₓ and Iᵧᵧ
Iₓₓ = ( Iₓₓ )₁ + ( Iₓₓ )₂ + ( Iₓₓ )₃
= [ 200x 203 / 12 + 4000 (10-200)2 ] + [ 200x 3603 / 12 + 1200 (200-200)2 ] + [ 200x 203 / 12 + 4000 (390-200)2 ]
= 366826666.7 mm4
Iᵧᵧ = ( Iᵧᵧ )₁ + ( Iᵧᵧ )₂ + ( Iᵧᵧ )₃
= [ 20x 2003 / 12 + 4000 (100-100)2 ] + [ 360 x 203 / 12 + 0 ] + [ 20x 2003 / 12 + 0 ]
= 26906666.67 mm4
Iᵧᵧ < Iₓₓ so, I = 26906666.67 mm4
Buckling Load (Pcr) = π2 EI / Le2
= π2 200 x 109 x 26906666.67 / 32
= 5901.289 KN
4. A hollow tube 4 m long with external & internal diameter 40 mm & 25 mm respectively was found to extend 4.8 mm under a tensile load of 60 KN. Find the buckling load for the tune with both ends pinned. Also, Find the safe load on the tube taking FOS as 5.
Given,
L= 4m
D= 40 mm
d= 25 mm
FOS = 5
Δl = 4.8 mm
Buckling load = ?
Both ends are pinned so, Le= L =4m
Buckling Load (Pcr) = π2 EI / Le2 …………(i)
Moment of Inertia (I) = π ( D4 – d4 ) / 64
= π ( 0.044 – 0.0254 ) / 64
= 1.6 x 10-7 mm4
E = FL/ AΔl
= ( 60 x 103 x 4 x 4 ) / (0.042 – 0.0252 ) x 4.8 x 10-3
= 6.53 x 1010 N/m2
From eqn (i),
Pcr = π2 6.53 x1010 x 1.6 x 10-7 / 42
=4270 N
Safe load = Buckling load / FOS
= 4270/5
Safe load = 854 N
5. Determine the ratio of buckling strength of 2 columns of circular cross-section one hollow and other solid when both are made of the same material, have the same length, same cross-sectional area, and same end conditions. The internal diameter of the hollow column is half of its external diameter.
Given,
| Hollow | Solid |
| Eᴴ | E |
| lᴴ | l |
| Aᴴ | A |
dᴴ = Dᴴ / 2
Buckling Load (Pcr)ᴴ = π2 Eᴴ Iᴴ / Le2 ………….(i)
Buckling Load (Pcr) = π2 E I / Le2 …………(ii)
(Pcr)ᴴ / (Pcr) = Iᴴ / I
= ( Dᴴ4 – dᴴ4 ) / D4
= 0.938 Dᴴ4 / D4 …………..(iii)
Since the cross-sectional area is same of both columns,
A = Aᴴ
π D2 / 4 = π Dᴴ2 / 4
Dᴴ2 / D2 = 1.333
Dᴴ / D = 1.1547
from (iii), we get
(Pcr)ᴴ / (Pcr) = 0.938 (1.1547)4
(Pcr)ᴴ / (Pcr) = 1.66
| Read More: Earned Value Analysis |
| Read More: Preliminary Estimate |


